what is movement in relation to a frame of reference.

April 07, 2022 Post a Comment

Abstract coordinate system and the set of concrete reference points that uniquely fix (locate and orient) the coordinate system and standardize measurement (s)

In physics and astronomy, a frame of reference (or reference frame) is an abstract coordinate organization with an origin, orientation, and calibration specified by a set of reference points―geometric points whose position is identified both mathematically (with numerical coordinate values) and physically (signaled by conventional markers).^[1]

For n dimensions, n + 1 reference points are sufficient to fully ascertain a reference frame. Using rectangular Cartesian coordinates, a reference frame may be divers with a reference point at the origin and a reference signal at ane unit altitude along each of the n coordinate axes.^{[ commendation needed ]}

In Einsteinian relativity, reference frames are used to specify the relationship between a moving observer and the phenomenon under ascertainment. In this context, the term oft becomes observational frame of reference or observational reference frame , which implies that the observer is at residuum in the frame, although not necessarily located at its origin. A relativistic reference frame includes (or implies) the coordinate fourth dimension, which does not equate beyond different reference frames moving relatively to each other. The situation thus differs from Galilean relativity, in which all possible coordinate times are essentially equivalent.^{[ citation needed ]}

Definition [edit]

The need to distinguish between the diverse meanings of "frame of reference" has led to a variety of terms. For case, sometimes the type of coordinate system is attached as a modifier, as in Cartesian frame of reference. Sometimes the state of motion is emphasized, as in rotating frame of reference. Sometimes the way it transforms to frames considered every bit related is emphasized equally in Galilean frame of reference. Sometimes frames are distinguished by the scale of their observations, as in macroscopic and microscopic frames of reference.^[2]

In this commodity, the term observational frame of reference is used when emphasis is upon the state of movement rather than upon the coordinate choice or the grapheme of the observations or observational apparatus. In this sense, an observational frame of reference allows study of the issue of motion upon an entire family of coordinate systems that could be attached to this frame. On the other hand, a coordinate system may be employed for many purposes where the state of motion is not the principal concern. For example, a coordinate organisation may be adopted to have advantage of the symmetry of a system. In a still broader perspective, the formulation of many problems in physics employs generalized coordinates, normal modes or eigenvectors, which are only indirectly related to infinite and time. It seems useful to divorce the various aspects of a reference frame for the give-and-take below. We therefore take observational frames of reference, coordinate systems, and observational equipment as independent concepts, separated as below:

An observational frame (such as an inertial frame or non-inertial frame of reference) is a physical concept related to country of motion.
A coordinate arrangement is a mathematical concept, amounting to a choice of language used to describe observations.^[3] Consequently, an observer in an observational frame of reference can choose to employ any coordinate system (Cartesian, polar, curvilinear, generalized, …) to depict observations made from that frame of reference. A alter in the choice of this coordinate organisation does not change an observer's country of motion, and and so does not entail a change in the observer'southward observational frame of reference. This viewpoint can be found elsewhere equally well.^[4] Which is not to dispute that some coordinate systems may be a better choice for some observations than are others.

Choice of what to measure and with what observational apparatus is a thing separate from the observer'southward state of motion and choice of coordinate system.

Hither is a quotation applicative to moving observational frames ${\mathfrak {R}}$ and various associated Euclidean three-space coordinate systems [R, R′, etc.]:^[5]

Nosotros first introduce the notion of reference frame, itself related to the idea of observer: the reference frame is, in some sense, the "Euclidean space carried by the observer". Permit us give a more mathematical definition:… the reference frame is... the gear up of all points in the Euclidean space with the rigid body motion of the observer. The frame, denoted ${\mathfrak {R}}$ , is said to move with the observer.… The spatial positions of particles are labelled relative to a frame ${\mathfrak {R}}$ past establishing a coordinate system R with origin O. The corresponding ready of axes, sharing the rigid torso move of the frame ${\mathfrak {R}}$ , tin be considered to requite a physical realization of ${\mathfrak {R}}$ . In a frame ${\mathfrak {R}}$ , coordinates are changed from R to R′ by carrying out, at each instant of fourth dimension, the same coordinate transformation on the components of intrinsic objects (vectors and tensors) introduced to correspond concrete quantities in this frame.

and this on the utility of separating the notions of ${\mathfrak {R}}$ and [R, R′, etc.]:^[6]

Equally noted by Brillouin, a distinction between mathematical sets of coordinates and physical frames of reference must be fabricated. The ignorance of such distinction is the source of much confusion… the dependent functions such as velocity for example, are measured with respect to a physical reference frame, but one is free to choose any mathematical coordinate arrangement in which the equations are specified.

and this, too on the distinction betwixt ${\mathfrak {R}}$ and [R, R′, etc.]:^[7]

The idea of a reference frame is really quite different from that of a coordinate organization. Frames differ just when they define unlike spaces (sets of rest points) or times (sets of simultaneous events). So the ideas of a space, a time, of rest and simultaneity, go inextricably together with that of frame. Still, a mere shift of origin, or a purely spatial rotation of space coordinates results in a new coordinate system. So frames represent at best to classes of coordinate systems.

and from J. D. Norton:^[8]

In traditional developments of special and general relativity it has been customary not to distinguish between ii quite singled-out ideas. The kickoff is the notion of a coordinate organisation, understood simply as the polish, invertible consignment of iv numbers to events in spacetime neighborhoods. The second, the frame of reference, refers to an arcadian system used to assign such numbers […] To avert unnecessary restrictions, we tin divorce this arrangement from metrical notions. […] Of special importance for our purposes is that each frame of reference has a definite land of motion at each event of spacetime. […] Within the context of special relativity and as long as nosotros restrict ourselves to frames of reference in inertial motion, then petty of importance depends on the difference between an inertial frame of reference and the inertial coordinate system it induces. This comfy circumstance ceases immediately once nosotros begin to consider frames of reference in nonuniform move even within special relativity.…More recently, to negotiate the obvious ambiguities of Einstein's treatment, the notion of frame of reference has reappeared as a structure distinct from a coordinate organisation.

The discussion is taken beyond unproblematic space-fourth dimension coordinate systems by Brading and Castellani.^[9] Extension to coordinate systems using generalized coordinates underlies the Hamiltonian and Lagrangian formulations^[ten] of breakthrough field theory, classical relativistic mechanics, and breakthrough gravity.^[11] ^[12] ^[13] ^[14] ^[xv]

Coordinate systems [edit]

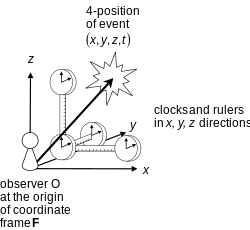
An observer O, situated at the origin of a local set of coordinates – a frame of reference F. The observer in this frame uses the coordinates (x, y, z, t) to describe a spacetime issue, shown as a star.

Although the term "coordinate system" is often used (particularly past physicists) in a nontechnical sense, the term "coordinate system" does accept a precise meaning in mathematics, and sometimes that is what the physicist means as well.

A coordinate system in mathematics is a facet of geometry or of algebra,^[xvi] ^[17] in particular, a property of manifolds (for example, in physics, configuration spaces or phase spaces).^[xviii] ^[xix] The coordinates of a point r in an north-dimensional space are but an ordered set up of n numbers:^[20] ^[21]

\mathbf {r} =[x^{i},\ x^{2},\ \dots ,\ x^{due north}].

In a full general Banach infinite, these numbers could be (for instance) coefficients in a functional expansion similar a Fourier serial. In a physical trouble, they could be spacetime coordinates or normal style amplitudes. In a robot blueprint, they could be angles of relative rotations, linear displacements, or deformations of joints.^[22] Hither we will suppose these coordinates tin be related to a Cartesian coordinate system by a set of functions:

x^{j}=10^{j}(10,\ y,\ z,\ \dots ),\quad j=ane,\ \dots ,\ n,

where x, y, z, etc. are the due north Cartesian coordinates of the point. Given these functions, coordinate surfaces are defined past the relations:

ten^{j}(x,y,z,\dots )=\mathrm {constant} ,\quad j=i,\ \dots ,\ n.

The intersection of these surfaces define coordinate lines. At any selected point, tangents to the intersecting coordinate lines at that point ascertain a set of footing vectors {e _i, east ₂, …, eastward _{due north}} at that betoken. That is:^[23]

\mathbf {e} _{i}(\mathbf {r} )=\lim _{\epsilon \rightarrow 0}{\frac {\mathbf {r} \left(x^{ane},\ \dots ,\ 10^{i}+\epsilon ,\ \dots ,\ x^{n}\right)-\mathbf {r} \left(x^{1},\ \dots ,\ x^{i},\ \dots ,\ 10^{due north}\right)}{\epsilon }},\quad i=ane,\ \dots ,\ n,

which can be normalized to exist of unit length. For more item see curvilinear coordinates.

Coordinate surfaces, coordinate lines, and basis vectors are components of a coordinate system.^[24] If the basis vectors are orthogonal at every betoken, the coordinate system is an orthogonal coordinate organization.

An important attribute of a coordinate organization is its metric tensor grand_ik , which determines the arc length ds in the coordinate arrangement in terms of its coordinates:^[25]

(ds)^{2}=g_{ik}\ dx^{i}\ dx^{grand},

where repeated indices are summed over.

Equally is apparent from these remarks, a coordinate organization is a mathematical construct, part of an axiomatic system. There is no necessary connection between coordinate systems and physical motion (or any other aspect of reality). All the same, coordinate systems tin can include fourth dimension equally a coordinate, and tin can exist used to describe motility. Thus, Lorentz transformations and Galilean transformations may be viewed equally coordinate transformations.

General and specific topics of coordinate systems can be pursued following the See too links below.

Physics [edit]

Three frames of reference in special relativity. The blackness frame is at residual. The primed frame moves at 40% of lite speed, and the double primed frame at 80%. Annotation the scissors-like change as speed increases.

An observational frame of reference, oft referred to as a concrete frame of reference, a frame of reference, or simply a frame, is a physical concept related to an observer and the observer's state of motion. Here we adopt the view expressed by Kumar and Barve: an observational frame of reference is characterized only by its country of move.^[26] However, there is lack of unanimity on this point. In special relativity, the distinction is sometimes fabricated betwixt an observer and a frame. According to this view, a frame is an observer plus a coordinate lattice constructed to exist an orthonormal correct-handed set of spacelike vectors perpendicular to a timelike vector. See Doran.^[27] This restricted view is not used hither, and is not universally adopted fifty-fifty in discussions of relativity.^[28] ^[29] In general relativity the utilize of full general coordinate systems is common (see, for example, the Schwarzschild solution for the gravitational field outside an isolated sphere^[30]).

There are 2 types of observational reference frame: inertial and non-inertial. An inertial frame of reference is divers every bit one in which all laws of physics have on their simplest grade. In special relativity these frames are related past Lorentz transformations, which are parametrized by rapidity. In Newtonian mechanics, a more restricted definition requires only that Newton'south first law holds true; that is, a Newtonian inertial frame is one in which a gratis particle travels in a straight line at constant speed, or is at residual. These frames are related by Galilean transformations. These relativistic and Newtonian transformations are expressed in spaces of full general dimension in terms of representations of the Poincaré group and of the Galilean group.

In contrast to the inertial frame, a non-inertial frame of reference is one in which fictitious forces must be invoked to explain observations. An example is an observational frame of reference centered at a point on the Globe'southward surface. This frame of reference orbits around the center of the Earth, which introduces the fictitious forces known as the Coriolis force, centrifugal force, and gravitational force. (All of these forces including gravity disappear in a truly inertial reference frame, which is one of free-fall.)

Measurement apparatus [edit]

A further aspect of a frame of reference is the role of the measurement appliance (for example, clocks and rods) attached to the frame (see Norton quote in a higher place). This question is not addressed in this article, and is of particular interest in breakthrough mechanics, where the relation between observer and measurement is all the same nether discussion (see measurement problem).

In physics experiments, the frame of reference in which the laboratory measurement devices are at balance is normally referred to as the laboratory frame or only "lab frame." An instance would be the frame in which the detectors for a particle accelerator are at rest. The lab frame in some experiments is an inertial frame, simply it is not required to be (for example the laboratory on the surface of the Earth in many physics experiments is non inertial). In particle physics experiments, it is often useful to transform energies and momenta of particles from the lab frame where they are measured, to the center of momentum frame "COM frame" in which calculations are sometimes simplified, since potentially all kinetic free energy however nowadays in the COM frame may exist used for making new particles.

In this connexion it may exist noted that the clocks and rods oft used to draw observers' measurement equipment in thought, in practise are replaced by a much more than complicated and indirect metrology that is connected to the nature of the vacuum, and uses atomic clocks that operate according to the standard model and that must exist corrected for gravitational time dilation.^[31] (Encounter second, meter and kilogram).

In fact, Einstein felt that clocks and rods were simply expedient measuring devices and they should be replaced past more central entities based upon, for case, atoms and molecules.^[32]

Instances [edit]

International Terrestrial Reference Frame
International Angelic Reference Frame
In fluid mechanics, Lagrangian and Eulerian specification of the flow field

Other frames

Frame fields in full general relativity
Moving frame in mathematics

Notes [edit]

^ Kovalevsky, J.; Mueller, Ivan I. (1989). "Introduction". Reference Frames. Astrophysics and Space Scientific discipline Library. Vol. 154. Dordrecht: Springer Netherlands. pp. one–12. doi:10.1007/978-94-009-0933-5_1. ISBN978-94-010-6909-0. ISSN 0067-0057.
^ The distinction betwixt macroscopic and microscopic frames shows up, for example, in electromagnetism where constitutive relations of diverse time and length scales are used to make up one's mind the current and charge densities entering Maxwell's equations. Encounter, for example, Kurt Edmund Oughstun (2006). Electromagnetic and Optical Pulse Propagation i: Spectral Representations in Temporally Dispersive Media. Springer. p. 165. ISBN0-387-34599-Ten. . These distinctions also appear in thermodynamics. Encounter Paul McEvoy (2002). Classical Theory. MicroAnalytix. p. 205. ISBN1-930832-02-8. .
^ In very general terms, a coordinate organisation is a prepare of arcs x ⁱ = x ⁱ (t) in a complex Lie group; encounter Lev Semenovich Pontri͡agin (1986). L.South. Pontryagin: Selected Works Vol. 2: Topological Groups (tertiary ed.). Gordon and Breach. p. 429. ISBN2-88124-133-6. . Less abstractly, a coordinate system in a space of n-dimensions is defined in terms of a footing prepare of vectors {e ₁, e ₂,… e _n}; meet Edoardo Sernesi; J. Montaldi (1993). Linear Algebra: A Geometric Approach. CRC Press. p. 95. ISBN0-412-40680-two. As such, the coordinate system is a mathematical construct, a language, that may exist related to movement, but has no necessary connection to motility.
^ J X Zheng-Johansson; Per-Ivar Johansson (2006). Unification of Classical, Quantum and Relativistic Mechanics and of the Four Forces. Nova Publishers. p. thirteen. ISBN1-59454-260-0.
^ Jean Salençon; Stephen Lyle (2001). Handbook of Continuum Mechanics: General Concepts, Thermoelasticity. Springer. p. 9. ISBN3-540-41443-half dozen.
^ Patrick Cornille (Akhlesh Lakhtakia, editor) (1993). Essays on the Formal Aspects of Electromagnetic Theory. World Scientific. p. 149. ISBN981-02-0854-v.
^ Nerlich, Graham (1994). What Spacetime Explains: Metaphysical essays on infinite and fourth dimension. Cambridge University Press. p. 64. ISBN0-521-45261-9.
^ John D. Norton (1993). Full general covariance and the foundations of full general relativity: 8 decades of dispute, Rep. Prog. Phys., 56, pp. 835-7.
^ Katherine Brading; Elena Castellani (2003). Symmetries in Physics: Philosophical Reflections. Cambridge University Press. p. 417. ISBN0-521-82137-1.
^ Oliver Davis Johns (2005). Analytical Mechanics for Relativity and Quantum Mechanics. Oxford University Printing. Affiliate 16. ISBN0-nineteen-856726-X.
^ Donald T Greenwood (1997). Classical dynamics (Reprint of 1977 edition past Prentice-Hall ed.). Courier Dover Publications. p. 313. ISBN0-486-69690-ane.
^ Matthew A. Trump; W. C. Schieve (1999). Classical Relativistic Many-Body Dynamics. Springer. p. 99. ISBN0-7923-5737-X.
^ A S Kompaneyets (2003). Theoretical Physics (Reprint of the 1962 2d ed.). Courier Dover Publications. p. 118. ISBN0-486-49532-9.
^ One thousand Srednicki (2007). Quantum Field Theory. Cambridge University Printing. Chapter 4. ISBN978-0-521-86449-vii.
^ Carlo Rovelli (2004). Breakthrough Gravity. Cambridge Academy Press. p. 98 ff. ISBN0-521-83733-2.
^ William Barker; Roger Howe (2008). Continuous symmetry: from Euclid to Klein. American Mathematical Guild. p. 18 ff. ISBN978-0-8218-3900-iii.
^ Arlan Ramsay; Robert D. Richtmyer (1995). Introduction to Hyperbolic Geometry . Springer. p. 11. ISBN0-387-94339-0. geometry axiom coordinate organization.
^ According to Hawking and Ellis: "A manifold is a infinite locally similar to Euclidean space in that it can be covered by coordinate patches. This construction allows differentiation to be defined, but does non distinguish between different coordinate systems. Thus, the only concepts defined past the manifold structure are those that are independent of the choice of a coordinate system." Stephen Due west. Hawking; George Francis Rayner Ellis (1973). The Big Scale Structure of Space-Fourth dimension. Cambridge University Press. p. xi. ISBN0-521-09906-4. A mathematical definition is: A continued Hausdorff space M is chosen an n-dimensional manifold if each bespeak of K is contained in an open set that is homeomorphic to an open fix in Euclidean due north-dimensional infinite.
^ Shigeyuki Morita; Teruko Nagase; Katsumi Nomizu (2001). Geometry of Differential Forms . American Mathematical Social club Bookstore. p. 12. ISBN0-8218-1045-6. geometry axiom coordinate system.
^ Granino Arthur Korn; Theresa Yard. Korn (2000). Mathematical handbook for scientists and engineers : definitions, theorems, and formulas for reference and review. Courier Dover Publications. p. 169. ISBN0-486-41147-8.
^ See Encarta definition. Archived 2009-10-31.
^ Katsu Yamane (2004). Simulating and Generating Motions of Human Figures. Springer. pp. 12–13. ISBNiii-540-20317-6.
^ Achilleus Papapetrou (1974). Lectures on General Relativity. Springer. p. five. ISBN90-277-0540-2.
^ Wilford Zdunkowski; Andreas Bott (2003). Dynamics of the Atmosphere. Cambridge Academy Printing. p. 84. ISBN0-521-00666-X.
^ A. I. Borisenko; I. E. Tarapov; Richard A. Silverman (1979). Vector and Tensor Analysis with Applications. Courier Dover Publications. p. 86. ISBN0-486-63833-ii.
^ See Arvind Kumar; Shrish Barve (2003). How and Why in Basic Mechanics. Orient Longman. p. 115. ISBN81-7371-420-7.
^ Chris Doran; Anthony Lasenby (2003). Geometric Algebra for Physicists. Cambridge University Printing. p. §v.2.2, p. 133. ISBN978-0-521-71595-9. .
^ For case, Møller states: "Instead of Cartesian coordinates we can obviously just as well employ full general curvilinear coordinates for the fixation of points in physical space.…we shall now introduce general "curvilinear" coordinates ten ⁱ in 4-space…." C. Møller (1952). The Theory of Relativity. Oxford Academy Press. p. 222 and p. 233.
^ A. P. Lightman; West. H. Press; R. H. Price; S. A. Teukolsky (1975). Trouble Book in Relativity and Gravitation . Princeton University Press. p. 15. ISBN0-691-08162-Ten. relativistic general coordinates.
^ Richard L Faber (1983). Differential Geometry and Relativity Theory: an introduction. CRC Press. p. 211. ISBN0-8247-1749-Ten.
^ Richard Wolfson (2003). Simply Einstein. W W Norton & Co. p. 216. ISBN0-393-05154-4.
^ See Guido Rizzi; Matteo Luca Ruggiero (2003). Relativity in rotating frames. Springer. p. 33. ISBN1-4020-1805-3. .